Circle Geometry: Anlge Measures Formed By Intersecting Lines

Figure 1

As I think about circles in a plane and intersecting lines, I can think of more triangle proofs and work with the angle and segment addition postulates. This will lead to both algebraical and geometric types of problems. By algebraic, I mean you will have to use a theorem or property to set up the equation. On the other hand, a geometric type of problem will consist of a proof of a stated given or already known theorem. Before getting into solving any problems, I should discuss the theorem of topic for the geometry article.

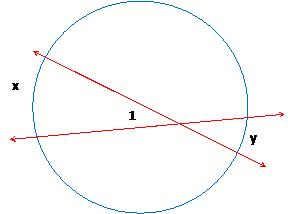
Theorem – Two Lines Intersecting Inside of a Circle

The measure of an angle formed by two lines that intersect inside a circle is half the sum of the measures of the intercepted arcs. Figure 1 illustrates the following equation to represent this theorem:

.

Example 1 – Find the measure of the missing angle measure.

The first example ends up being a an easy three step equation to solve.

Example 1

To set up the equation I HAVE to use geometry, in this case, Two Lines Intersecting Inside of a Circle Theorem (TLIICT), then I solve the equation using an algebra 1 skill.

.  TLIICT

(1). Substitution Property of Equality

(2) Multiplication Property of Equality

(3)  Subtraction Property of Equality

(4) Symmetric Property of Equality

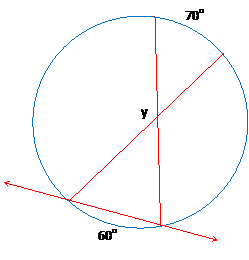
Example 2 – Find the measure of the missing angle measure.

Example 2

The solution to this problem may not present itself right away if one too focused on the current theorem. There is no variable for what you have to solve for first. To find the value of the variable, I must keep in mind that the measure of the angle I am solving for is supplementary to the measure of the angle I can find. Since it is not labeled in the original picture, in figure 2, I label what I can find with what is given, x.

Figure 2

Let x = to the value of the vertical angles formed not labeled y

In this post, I have discussed the Lines Intersecting Inside of a Circle Theorem and worked out two problems. I worked out the problems differently in each example. Which method do you prefer to read and why? I look forward to your answers.