Quadratic Functions and Their Graphs

Standard Form of a Quadratic Function

Where

The restriction on the quadratic term, , ensures that the function is indeed a quadratic function. Without the squared term, you are left with a linear function or a constant function.

A simple skill assessed in most high school algebra 2 classes is the ability to classify functions. If you are studying quadratics, then you have studied linear equations with their close relative the constant function. To classify a function, it is often necessary to simplify what is given in order to properly determine the type of function.

Example 1 Identifying Functions

Classify each equation as linear or quadratic and identify the quadratic, linear and constant terms.
(1)

(2)

(3)

(4)

In the steps above, line 1 represents the given function. Line 2 represents simplifying the equation with multiplication. You may have heard of the acronym FOIL, which stands for: First, Outer, Inner, Last. This is a short cut to multiplying to binomials. Anyway, line 3 is formed by actually performing the multiplication set up in line 2. The change from line 3 to 4 occurs by collecting or adding the like terms of -4x and -6x to give the linear term -10x. The quadratic term is and the constant term is .

Example 2 Understanding the Graph of Parabola

As with working with linear functions, the ability to interpret and analyze the graphs of basic quadratic functions is critical. It is good to note that the graph of a quadratic function is called a parabola. Figure 1 is the graph to the parabola, . For now, the function in the previous sentence is irrelevant. The reason for this, the graph is given. I will discuss in an upcoming post how the actual function is related to the graph of the related parabola.

Figure 1 Parts of a Parabola

In figure 1, is graphed and has vertex of and an axis of symmetry (AOS) of . Though I did not label the other two distinguishable points, you should be able to see, that the other points on parabola correspond to each other. This is because parabolas are symmetric, hence the axis of symmetry. Those points are equidistant from . Since this parabola opens upward, it has a minimum or bottom value. You get the value from the y-coordinate. The reason you use the y-coordinate is there is not bound on the Domain. Parabolas extend in the both directions along the x-axis. Because of the shape of parabola, there is a limit on the range. In this case, the range could be expressed as {y|y≥-4}.

The previous paragraph can be boiled down to identifying the AOS, vertex, points and corresponding points on the graph of a quadratic function or parabola.

Figure 2 Parts of a Parabola

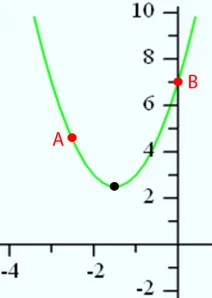
Example 3 Understanding the Graph of Parabola For you to do.

For figure 2, identify the axis of symmetry, the vertex, the minimum or maximum value and explain why it is a min or a max value, identify both points and their mirror image about the AOS.

Click here for the solution.

Parabolas – Identifing Parts of a Parabola

Example 3 Understanding the Graph of Parabola For you to do.

Identifying Parts of a Parabola

For the figure, identify the axis of symmetry, the vertex, the minimum or maximum value and explain why it is a min or a max value, identify both points and their mirror image about the AOS.

The axis of symmetry is . Since the parabola is facing up the y-coordinate of the vertex represent a minimum value. The vertex is . The minimum value is .
Point A is and its corresponding point would be
Point B is and its corresponding point would be

Return to the original post: click here.